Kerala PSC HSST Mathematics Solved paper Part 4
Paper Code: 44/2017/OL
Category Code: 315/2015
Exam: HSST(Jr) Mathematics NCA
Date of Test 02-06-2017
HSST Mathematics detailed syllabus: Click here
A:-`T(x,y,z)=5`
B:-`T(x, y, z) = x^(2)`
C:-`T(x,y,z)=-2x+y`
D:-`T(x,y, z)=xy+6`
Correct Answer:- Option-C
76:-Let V be a vector of dimension 15 over a field F and W be a subspace of V. If dim W = 3, then `dim(V//W)=`
A:-3
B:-5
C:-8
D:-12
Answer:- Option-D
77:-An `nxxn` matrix is diagonalizable if
A:-all the eigen values are real and distinct
B:-all the eigen values are real and non-negative
C:-all the eigen values are real and non-zero
D:-all the eigen values are non-zero rational numbers
Answer:- Option-A
78:-Let `1<=p<r<oo` and let `x inl^(p)` with `||x||_(p)<=1` . Then the inequality `||x||_(r)<=||x||_(p)` is called
A:-Minkowski's inequality
B:-Jensen's inequality
C:-Cauchy's inequality
D:-Bessel's inequality
Answer:- Option-B
79:-Which of the following is false ?
A:-`l^(p)` is a Hilbert space, where `1<=p<=oo`
B:-Closed subspace of a Hilbert space is a Hilbert space
C:-The quotient of a Hilbert space by one of its closed subspace is again a Hilbert space
D:-A complete normed space with its norm satisfies the parallelogram law is a Hilbert space
Answer:- Option-A
80:-Let A`in`BL(H) and A* be the adjoint of A. A is unitary if
A:-AA* = A*A
B:-AA* `!=` A*A
C:-A* = `A^(-1)`
D:-A* = A
Answer:- Option-C
81:-Which of the following is not a Banach space ?
A:-Finite dimensional normed spaces
B:-`l^(p)` with norm `||` `||_(p)`, where `1<= p<=oo `
C:-`C_(00)`
D:-`L^(p)(E)` with the norm `||` `||_(p)`, where E is a Lebesgue measurable subset of `RR` and `1<=p<=oo`
Answer:- Option-C
82:-Let X be an inner product space and let x, y `in` X. Then the parallelogram law is
A:-`||x+y||^(2)+||x-y||^(2)=2(||x||^(2)+||y||^(2))`
B:-`||x+y||^(2)+||x-y||^(2)=||x||^(2)+||y||^(2)`
C:-`||x+y||<=||x+z||+||z+y||`
D:-`sqrt(||x||^(2)+||y||^(2))<=||x+y||`
Answer:- Option-A
83:-Let X and Y be normed spaces and F : X `->` Y be a linear map. The following conditions are equivalent except
one. Which of the following is not equivalent to others ?
A:-F is continuous at 0
B:-F is continuous on X
C:-`a||x||<=||F(x)||`, for all x`in`X and some a > 0
D:-F is uniformly continuous on X
Answer:- Option-C
84:-If a, b, c are the roots of the equation `x^(3)+px^(2)+qx+r=0`, then `1/a+1/b+1/c=`
A:-`(-q)/(r)`
B:-`(p)/(r)`
C:-`(-p)/(r)`
D:-`p/q`
Answer:- Option-A
85:-
86:-`(d)/(dx)sinh^(-1)(x)=`
A:-`(1)/(sqrt(1-x^(2)))`
B:-`(1)/(sqrt(1+x^(2)))`
C:-`(1)/(sqrt(x^(2)-1))`
D:-`(1)/(1+x^(2))`
Answer:- Option-B
87:-Equation of the tangent at the point `(x_(1),y_(1))` on the parabola `y^(2)=4ax` is
A:-`yy_(1)=2a(x+x_(1))`
B:-`y-y_(1)=4a(x-x_(1))`
C:-`y=y_(1)/x_(1)(x-2a)`
D:-y = 2ax
Answer:- Option-A
88:-Rank of the matrix `[[3, 4, 1], [-2, 3, 2], [5, 1, -1]]` is
A:-0
B:-1
C:-2
D:-3
Answer:- Option-C
89:-`lim_((x,y)->(0,0)` `((5x^(2)y)/(2x^(4)+y^(2)))=`
A:-0
B:-`5/3`
C:-`5/2`
D:-limit does not exist
Answer:- Option-D
90:-Let `f(x)=x^(3)-12x+9`. Then f(x) has a local maximum at
A:-x = 0
B:-x = 3
C:-x = 2
D:-x = –2
Answer:- Option-D
91:-Let `W=x^(2)+y^(2)` , x = r – S and y = r + S. Then the partial derivative of W with respect to S is
A:-`(delW)/(delS)=1`
B:-`(delW)/(delS)=2S`
C:-`(delW)/(delS)=4S`
D:-`(delW)/(delS)=2r`
Answer:- Option-C
92:-Solution of the differential equation `(d^(2)y)/(dx^(2))-6dy/dx+13y=0` is
A:-`Ae^(3x)+Be^(2x)`B:-`e^(3x)(Acos2x+Bsin2x)`
C:-Acos2x + Bsin2x
D:-`Ax^(2)+Bx^(3)`
Answer:- Option-B
93:-Solution of the equation `(1+2xy+y^(2))dx+(1+2xy+x^(2))dy=0` is
A:-`x+x^(2)y+xy^(2)+y=k`
B:-`x+2x^(2)y+2xy^(2)+y=k`
C:-`4x+4y=k`
D:-`1+2xy+x^(2)+y^(2)=k`
Answer:- Option-A
94:-Let `f(x)=sum_(n=1)^oob_(n)sinnx` be the Fourier series of f(x) = x in the interval `[-pi, Pi]` . Then `b_(n)=`
A:-0
B:-`(1)/(n)`
C:-`(-1)^(n)/(n^(2))`
D:-`(2(-1)^(n+1)^)/(n)`
Answer:- Option-D
95:-Laplace transform of `e^(at)sinbt` is
A:-`(s)/(s^(2)+b^(2))`
B:-`(s-a)/((s-a)^(2)+b^(2))`
C:-`b/((s-a)^(2)+b^(2))`
D:-`b/((s-a)^(2)-b^(2))`
Answer:- Option-C
96:-Two dimensional Laplace equation is
A:-`(del^(2)u)/(delt^(2))=c^(2)(del^(2)u)/(delx^(2))`
B:-`(del^(2)u)/(delx^(2))+(del^(2)u)/(dely^(2))=0`
C:-`(del^(2)u)/(delx^(2))+(del^(2)u)/(dely^(2))=f(x,y)`
D:-`(delu)/(delt)=c^(2)(del^(2)u)/(delx^(2))`
Answer:- Option-B
97:-Value of the Beta function at `(1/2,1/2)` is
A:-`beta(1/2,1/2)=Pi`
B:-`beta(1/2,1/2)=sqrt(Pi)`
C:-`beta(1/2,1/2)=(Pi)/(2)`
D:-`beta(1/2,1/2)=1`
Answer:- Option-A
98:-Value of the Riemann Zeta function `zeta`(s) at s = 2 is
A:-`zeta(2)=1`
B:-`zeta(2)=2!`
C:-`zeta(2)=Pi/2`
D:-`zeta(2)=(Pi^(2))/(6)`
Answer:- Option-D
99:-Let T, N, B and k be unit tangent vector, principal unit normal vector, binormal vector and curvature respectively. Then
Answer:- Option-A
100:-Let A and B be fuzzy subsets of a crisp set X. If `mu_(A)(x)` and `mu_(B)(x)`` ` are the membership value of x
in A and B respectively, then which of the following gives a membership value of x in `AnnB`
A:-`max{mu_(A)(x), mu_(B)(x)}`
B:-`mu_(A)(x)+ mu_(B)(x)-mu_(A)(x)mu_(B)(x)`
C:-`min{mu_(A)(x), mu_(B)(x)}`
D:-`1-mu_(A)(x)mu_(B)(x)`
Answer:- Option-C

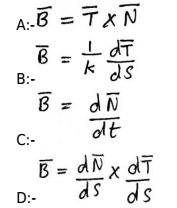

0 comments
Post a Comment